
Minimal geometries for magnetically actuated artificial microswimmers
Development of artificial microswimmers capable of propulsion in the low Reynolds number microscale environment has been pursued for micromanufacturing, drug delivery, and sensing applications. For microrobots actuated by a rotating magnetic field, previous research has focused on relatively complex flexible or chiral geometries for locomotion in bulk fluid. Recently, collaborator MinJun Kim’s group (Drexel University) has fabricated rigid, achiral magnetically actuated microswimmers. We have performed a symmetry analysis to understand the minimum geometric requirements for the design of such microrobots capable of low Reynolds number propulsion (published in Phys. Rev. E pdf). [Funded by NSF CBET-1067798]
Top image caption: An achiral, rigid magnetically actuated swimmer constructed of 3 spherical beads (Kim group, Drexel University). Second image caption: The three-bead swimmer is rotated by a external rotating magnetic field.
Bistability and control of minimal achiral magnetic microswimmers
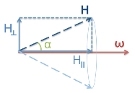
To model the rotational and swimming dynamics of the achiral swimmer, we developed a method of analyzing the magnetically-induced rotation of any rigid geometry. For the achiral three-bead swimmer, we found that there are regions of bistability where more than one rotation axis can be observed for the same magnetic field strength and rotation frequency. We show how altering the angle between the magnetic field and its rotation axis can be used to control bistability and the swimming direction of such swimmers.
Bistability has also been observed for helical microswimmers, and these results suggest possible applications to other geometries as well. [Funded by NSF CBET-1067798]
Image caption: The angle between the rotating magnetic field and rotation axis can be used to control bistability and swimming direction of three-bead swimmers.
Optimal moments and geometries for magnetically rotated helical microswimmers
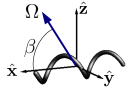
So far, the most common magnetically rotated microswimmers that swim in bulk fluid are helical. We model the full rotational and swimming dynamics of a helical geometry to investigate optimal magnetic moments and geometries. To achieve precise control, a linear relationship between rotation frequency and velocity is desirable. We find moments which guarantee such linear response, and helical geometries that optimize swimming velocities for such moments. [Funded by NSF DMR-1307497 and CMMI-1435652]
Image caption: A magnetically rotated helix will have a linear velocity-frequency response if the rotation axis is constant as frequency changes.

Shepherding swarms of magnetic microswimmers
In collaboration with Jake Abbott (University of Utah), we are investigating how nonuniform dipolar magnetic fields, swimmer-by-swimmer variability in response, and nonlinear response to magnetic field rotation rates can be used to control swarms of microswimmers. The goal is to develop control methods to steer, group, and separate groups of swimmers that do not require individual-level feedback of microswimmers. [Funded by NSF CMMI-1435652]
Image caption: How can you control a swarm of microswimmers without individual-level feedback, such as in vivo, where visualization is difficult?

Modular microrobotics with magnetic microswimmers
Modular systems of swimming microrobots that can change configuration to respond to changes in environment or to achieve different tasks may be particularly useful for in vivo applications such as drug delivery, diagnostics, or microsurgery, where microrobots encounter a wide array of environments. In collaboration with MinJun Kim, we are building off our previous work that showed that simple geometries are capable of propulsion, to develop microswimmers with geometries suitable to act as modular subunits. Currently, we are investigating how combined magnetic and hydrodynamic interactions can be used to aid the directed assembly of modules.
Image caption: As it swims downwards, magnetic and hydrodynamic interactions make magnetic particles in the zone below the swimmer likely to assemble with the swimmer.






